the case for scales (and the circle of 5ths)

In your adventures on this site, you will most likely observe that I emphasize scales a lot more than most guitar instructors. There are very good reasons for this. Music is a language. The chromatic scale is the alphabet. Major scales are your first words. They provide the context for everything else you're going to learn. They are the basis of the entire vocabulary of music. What they do for your technique can't even be measured. You'll gain so much in the way of control and tone that it will more than offset the hours you spent practicing them. Everything I’ve written about below should be cross-referenced with the material in Lessons Four, Nine, Twelve, Sixteen, and Eighteen.

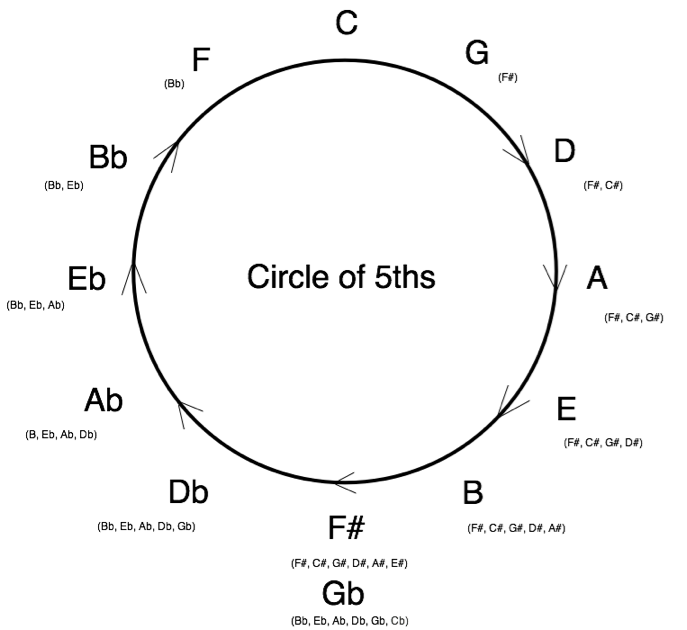
We’re going to be talking a lot about “keys” here. A key is basically the scale that a piece of music is based upon. On this site we work our way through all of the sharp keys, starting from C and working our way through the Circle of 5ths until we reach the Key of F#. Following the Circle of 5ths means that every new scale we learn is going to start at the 5th note of the last. For example, our first scale is C. The 5th note of the C scale is G, which is why G is the second scale we learn. The 5th note of the G scale is D, which will be the third scale in the sequence. Starting from D and counting up five leads to A, the fourth scale we learn. And so it goes.

Here's the interesting part. Every time we go up a 5th we get a new sharp. Each new scale will contain whatever sharps the last one had, plus a new one. In keeping with the theme the new sharp will be five notes up from the last one added. Try it. The G scale (the first key to contain any sharps) has one sharp, namely F#. The D scale is the next in the series, and it holds on to the F#, and adds a C#. Note that the distance between F# to C# is five letters (F#-G-A-B-C#). The next scale in the series is A which maintains both the F# and the C# and adds a G# (C#-D-E-F#-G#). Another interesting thing to take note of is that in each scale the new sharp is the second to last note in the scale, which is the letter right before the root. In G, it's F#. In D it's C#, in A it's G#. Pretty cool stuff.

You may be wondering what happens when you run out of sharps. After all, only five letters in the musical alphabet use them. Scroll to the diagram below which looks like an eye chart for the chronically demented and look closely at the key of F#, which is located at the 6 o'clock position. You’ll see that it has six sharps, the last being E#. If you’ve gone through Lesson Four you should be thinking that there is no such thing as E#, and you’re basically right in the sense that if you raise E by a half-step you get an F. In the case of the key of F#, however, we have to use the name E#, because every letter needs to be used in a major scale. We can’t use the name F because the key is F#, and you cannot use a letter twice. The reason for this is that written music uses a thing called a "key signature", which is a collection of #'s or b's at the beginning of the piece telling you in advance what notes are played sharp or flat. For example, at the very beginning of a song in the key of D you will see #’s on the F line and C space, because those are the two notes that are played sharp in that scale. A song in the key of B will have five, F#, C#, G#, D#, and A#. This is why we have to use an E# in the Key of F#, because you can't tell someone that all F's are to be played sharp when they're not. By using E# instead of F you have no redundancy. All E's are to be played a half-step higher, period.

Remember a couple of paragraphs ago when I said that every time you begin a 5th higher than the previous scale you get a new sharp? Try that starting from F# and you end up with C#. In accordance with the concepts laid out in the previous paragraph the Key of C# which will contain all #'s, including not only an E# but also a B#. Nobody wants to deal with that, so what we do is use the name Db instead of C#. Same sound, different name. By doing this we end up with the following scale:

Db-Eb-F-Gb-Ab-Bb-C-Db

As you can see, by calling the note Db instead of C# we're following all of the rules. Every letter is used, and we don't have any goofy notes (E#, B#, Fb, Cb). Much better. If we continue clockwise around the scale in this fashion we begin to shed flats. True to form, each flat we lose is a 5th higher than the last, until you wind up back at the 12 o’clock position, and the Key of C.